By Mike Travis
Recently, I spent some time in the Po‘e Ka‘ahele (fourth and fifth-grade) classroom at Hanahau‘oli School. The teacher shared with her students: “Today, we are learning more about a volunteer project we are working on to clean up the Ala Wai Canal.” She further described how the project involved making mud balls called, “Genki Balls,” out of dirt and “good” bacteria. When the Genki Balls are dried and thrown into the canal, they sink into the sludge at the bottom and release the bacteria, which will “eat” the sludge and make the Ala Wai cleaner for fish and humans. (It is fascinating to see how groups of people can work together to make the world a better place. If you want to learn more, click here.)
The teacher then shared, “Working together over an hour and a half, we will make about 300 Genki Balls. By the end of February 2024, volunteers had already made 115,000 Genki Balls, but their goal is to have produced and thrown in 300,000 Genki Balls by 2026, which should make the Ala Wai Canal safe for swimming.”
My mind was reeling as I thought about the numbers. If there are 60 people (students and teachers) working together for one hour and a half, and they produce 300 Genki Balls, how long does it take one person to produce one Genki Ball? And, how many Genki Balls do they still need to make to reach their goal? Could one person work 40 hours a week until 2026 and produce all the Genki Balls needed? Or, could a team work a couple of hours a week and finish on time?
I love math. I also love teaching math. For me, math is all around us. It is in everything we do, and no matter what school you teach at, we need to help students see this. The way we get better at math is by doing math, by asking questions and sharing our wonderings. My main takeaway for progressive educators looking to get students more engaged in math is to make math visible, accessible, and relevant to everyday experiences and problem-solving.
After teaching mathematics to students for more than 20 years, I think about it in terms of three strands: fluency, conceptual understanding, and application. Fluency is the ability to solve standard arithmetic problems (addition, subtraction, multiplication, and division) quickly and accurately. It usually involves procedural math like the standard algorithms. Conceptual understanding is the ability to comprehend the “why” of mathematics. It involves number sense, visually representing a problem and answer in different ways, and being able to explain why an answer is correct. Application is the ability to use mathematics for real-world problem-solving, for example, my Genki Ball questions at the start of the article.
In many elementary schools, the focus is primarily on fluency, memorizing the “times tables” and being able to solve long-division problems. It is about teaching the standard algorithms and making students practice them over and over again without any real understanding of why the algorithm works. This causes some students to become lost and disenchanted with math, especially students who have learning difficulties with memorization. In my opinion, math should never be about who can solve it fastest; it should be about asking:
What patterns do we see in this problem?
How many different ways can we approach a problem?
Can we estimate the answer before we begin?
How can we explain and justify our answers? Why are our answers reasonable?
Where might the problem lead us next?
How can math questions and answers be applied to the world around us?
I am not saying that you should spend all math class just chatting about numbers, although that does sound fun! If you notice the foundational triangle I created above, it is equilateral and cut into three equal areas. This is done on purpose. While some might think an integrated or project-based approach to teaching math must abandon the fluency portion for all conceptual learning, a balanced approach of all three will meet students where they are and ensure individual needs are met. While the tenets of a progressive educational philosophy are embodied in the ideas of “learning by doing,” “creating a community,” and “focusing on problem-solving,” there are times when giving direct instruction–even in math–is the best way to impart knowledge. Remember, it should be balanced in its components.
At Hanahau‘oli School, we’ve applied these ideas to our progressive education math program. We spent a year working together on a committee reviewing the national standards and deciding which benchmarks we wanted for each grade level. The benchmarks helped guide our planning and decision-making about how best to integrate math within our integrated thematic units of study. We realized that even in a progressive school, there is still a need for direct instruction for some benchmarks, but this can involve many approaches. Ways we can help to round out a student’s math education include small group program solving, skills practice, conceptual understanding (ideas from Jo Boaler), and even independent practice (homework). However, the focus of all math instruction must be on taking students where they are now and moving them forward with their mathematical knowledge. When teachers can connect math to a student’s passions, this leads to true success.
To wrap up these reflections on math teaching and learning in a progressive education setting, I share the following tips for teachers and parents.
Tips for Teachers
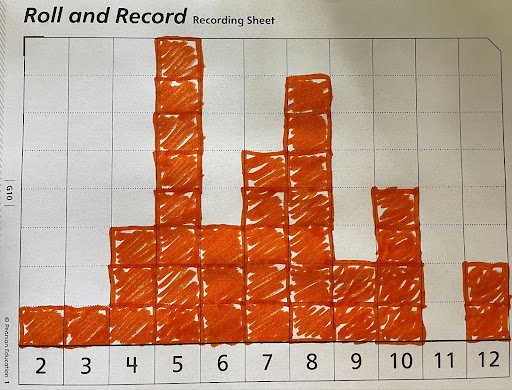
An example of "Roll and Record." Students roll a pair of dice and find the sum. They mark it on the sheet until one sum "wins.
Younger students love to engage in mathematics, especially when there is a little bit of a “gaming” aspect to it. Last year, I would visit our Kindergarten/First Grade mixed classes for what I called “math stations.” The goal was to engage the students in math, either related to the current unit of study or to the benchmarks we set for those grade levels. Generally, students would work in pairs; a Kindergarten and First Grade pairing allowed them to help each other with the task. One task that I observed almost all levels of math learners enjoy is “roll and record” sheets. In this station, paired students would take turns rolling the dice and recording the sum. The results vary, of course, but usually sums of six, seven, and eight come up the most, so this is something all the students can share together in a reflection at the end. (Need more of a challenge, create sheets for rolling 8-sided, 10-sided, 12-sided, or 20-sided dice. The sums will be bigger and students can predict the “winning” number. Older students will love this as an introduction to probability and looking at potential outcomes.)
Don’t forget the importance of discussion with students! Reflect on the math class or the activity at the end as a group. What went well? What didn’t go well? What would you do differently if you did this activity again? Also, give time for students to share the different ways they approached a problem. The way they approached and solved a problem can sometimes be more valuable than the actual answer. Another big question I ask students is if their answer is reasonable. Understanding how to estimate is critical in the real world as we don’t always have time to figure out the exact solution.
Tips for Parents
Remember that your children often reflect your attitudes and dispositions. So, even if you had a bad experience with math in your own schooling, do your best to have a positive outlook about mathematics in your home. Your attitude about math will affect your children’s future beliefs about it. Here are just a few examples our math committee created for parents and how you can work with children at home to engage in mathematical thinking:
Math is all around us - ask your child questions! How many flowers do you think are on that tree? How many lei do you think we could make from the flowers?
Show your child receipts from a store, and teach them about sales tax. Teach them to estimate what something actually costs.
Play games to practice math facts or greater than/less than games. Card games like War or Flashcards are fun for kids and parents as well.
Baking and cooking are fun and practical ways to practice budgeting (buying ingredients) measuring (amounts, temperature, converting recipes), and estimating (serving sizes, time needed).
Sorting objects is a great way for students to practice their math skills. Whether it is sorting markers, M&M candies, or coins, this will allow you to have a great discussion with your child about the results and their observations. Questions you can ask:
Which coin (marker color) had the most?
Which coin (marker color) had the least?
How many different coins (markers) are there?
How many altogether?
Students can also create a bar chart showing the data if they want.
Can’t figure out dinner as a family? Ask your child to help with the process by gathering data on what everyone in the family wants to eat. Your child can share the data with the family and “reveal” the top choice!
Allow your child independence involving math
Send your child to the counter to order and pay for food (students will learn the value of money, how to estimate, and how to independently ask for what they want)
Give your child money in a store to pay for a gift for Mother’s Day or a birthday gift. Allow your child to search and determine what gifts they can afford within the budget
Play games at home using money and giving change. (Pay Day and Monopoly are great examples.)
When at the store, find jars of items and ask them to guess how many items are in the jar. Then, use the ingredients list to find out the exact amount. (In this example, there are about 32 pieces in each ounce. Because there are 62 ounces in the whole container that would be 32 x 62 = 1,984 M&Ms in the whole container (approximately).
Tracking Distances
Using a Fitbit, iPhone, or some other GPS device, have your child track distances to the local park, the library, or to school. Ask them questions like: Do you think it is farther for us to walk to the park or the library? They could track the distances, graph them, and discuss the results. They could also track the number of steps they walk in a day. Why is the number so different on a weekday versus a weekend?
Going on a road trip on the mainland? Have your child help with plotting out the course, measuring the distances each day, and learning more about maps.
Hopefully, these reflections and tips on teaching math from a progressive educator’s perspective are helpful. As I shared at the beginning of the blog post, math is all around us. It is in everything we do. No matter what school you teach at, or how your own experiences were with math as a child, we need to help students see the value of math education. The way we get better at math is by doing math, by asking questions and sharing our wonderings. How will you make math visible, accessible, and relevant to everyday experiences and problem-solving?
Oh, by the way, in case you are interested, this is how I answered the questions from above:
How long does it take one person to produce one Genki Ball?
If 60 people work for 1.5 hours and make 300 Genki Balls, we can divide to figure out how long it takes (approximately) to make one ball.
300 ➗60 = 5 balls per person
1.5 hours x 60 minutes = 90 minutes work time
90 minutes ➗5 balls per person = 18 minutes per person to make one Genki Ball
How long will it take to meet their goal of producing and throwing 300,000 Genki Balls in the Ala Wai Canal by 2026?
Now, the organization wants to produce 300,000 Genki Balls. They had produced 115,000 balls already, so they still needed to make 185,000 (300,000 - 115,000) balls.
The organization had already produced 115,000 balls by the end of February 2024. They have until the end of 2025 (beginning of 2026) to finish 185,000 balls.
This gives them 10 months in 2024 and 12 months in 2025, or 22 months altogether. Let's say, there are 4 weeks every month, then: 22 months x 4 weeks = 88 weeks
If one person worked 40 hours a week for 88 weeks, how many Genki Balls could they produce?
Well, it takes 18 minutes per Genki Ball, so in one week, the person could produce: 40 hours x 60 minutes = 2400 minutes per week
2400 minutes ➗18 minutes ≈ 133.3 Genki Balls per week
In 88 weeks, that would be: 133.3 balls x 88 weeks = 11,730.4 Genki Balls
Oh, no, this is well short of the 185,000 Genki Balls needed!
What if you could get a team of volunteers who would work 20 hours a week to make Genki Balls? How many volunteers would you need to reach 185,000?
20 hours x 60 minutes = 1,200 minutes
1200 minutes ➗18 minutes ≈ 66.67 Genki Balls per week for one person
In 88 weeks, that would be: 66.67 x 88 weeks = 5,866.96 Genki Balls
So, to reach our goal of 185,000 Genki Balls, we would need:
185,000 ➗5866.96 ≈ 31.5 people --- Or, you would need 32 people working 20 hours per week, every week, to reach the goal of 300,000 Genki Balls by the end of 2025.
After solving this work, I would ask students if they had any other wonderings that we could investigate. Here are some of my wonderings:
What materials are needed to make a Genki Ball? And, if we want to make 185,000 more balls, how are we going to obtain all the materials?
Has the foundation considered having a big “all in one weekend” event? What if you could get a couple thousand people down to the Ala Wai Canal to work all weekend? Could you produce enough to finish the goal?
Is there a way to speed up the process? One of the limitations is the 18 minutes needed to create one Genki Ball. What if you could produce the balls in just five minutes?
Is there another way to get the “good” bacteria down to the sludge? (This starts to get into the “science” behind what the organization is doing and can lead students down a whole new path!)
ABOUT THE AUTHOR:
Dr. Mike Travis is the Director of Faculty and Curriculum, Upper Elementary at Hanahauʻoli School, where he has supported teachers since 2019. A passionate, lifelong learner, Mike has been an educator for more than 20 years working with students from elementary to graduate levels. Mike believes, "Educators truly can make a difference in the lives of their students, so I always serve with a strong sense of responsibility for their success and an inner energy and enthusiasm that are contagious to all around me." Mike loves long-distance running, spending time with family, and writing books.
Mike is moving to Hawaiʻi Island in July where he will return to teaching high school math and will pursue his dream of becoming a farmer with his wife in a two-acre forest in Keaʻau.